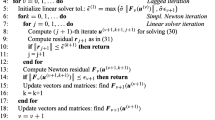Abstract
In this paper, we investigate a scheme for nonlinear reaction-diffusion equations using the mixed finite element methods. To linearize the mixed method equations, we use the two-grid algorithm. First, we solve the original nonlinear equations on the coarse grid, then, we solve the linearized problem on the fine grid used Newton iteration once. It is shown that the algorithm can achieve asymptotically optimal approximation as long as the mesh sizes satisfy \(H=\mathcal{O}(h^{\frac{1}{2}})\). As a result, solving such a large class of nonlinear equations will not much more difficult than the solution of one linearized equation.
Similar content being viewed by others
References
Arbogast, T., Wheeller, M.F., Yotov, I.: Mixed finite elements for elliptic problems with tensor coefficients as cell-centered finite differences. SIAM J. Numer. Anal. 32, 828–852 (1997)
Brezzi, F., Douglas, J. Jr., Marini, L.D.: Two families of mixed finite elements for second order elliptic problems. Numer. Math. 47, 217–235 (1985)
Chen, Y., Huang, Y.: A multilevel method for finite element solutions for singular two-point boundary value problems. Nat. Sci. J. Xiangtan Univ., 16, 23–26 (1994) (in Chinese)
Chen, Y., Li, L.: L p error estimates of two-grid schemes of expanded mixed finite element methods. Appl. Math. Comput. 209, 197–205 (2009)
Chen, Y., Huang, Y., Yu, D.: A two-grid method for expanded mixed finite-element solution of semilinear reaction-diffusion equations. Int. J. Numer. Methods Eng. 57, 193–209 (2003)
Chen, Y., Liu, H., Liu, S.: Analysis of two-grid methods for reaction-diffusion equations by expanded mixed finite element methods. Int. J. Numer. Methods Eng. 69, 408–422 (2007)
Chen, Y., Luan, P., Lu, Z.: Analysis of two-grid methods for nonlinear parabolic equations by expanded mixed finite element methods. Adv. Appl. Math. Mech. 1, 1–15 (2009)
Douglas, J. Jr., Roberts, J.E.: Mixed finite element methods for second order elliptic problems. Mat. Appl. Comput. 1, 91–103 (1982)
Douglas, J. Jr., Roberts, J.E.: Global estimates for mixed finite element methods for second order elliptic equations. Math. Comput. 44, 39–52 (1985)
Douglas, J. Jr., Ewing, R.E., Wheeler, M.F.: The approximation of the pressure by a mixed method in the simulation of miscible displacement. RAIRO. Anal. Numèr. 17, 17–33 (1983)
Dawson, C.N., Wheeler, M.F.: Two-grid method for mixed finite element approximations of non-linear parabolic equations. Contemp. Math. 180, 191–203 (1994)
Dawson, C.N., Wheeler, M.F., Woodward, C.S.: A two-grid finite difference scheme for nonlinear parabolic equations. SIAM J. Numer. Anal. 35, 435–452 (1998)
Garcia, M.F.: Improved error estimates for mixed finite element approximations for nonlinear parabolic equations: the continuously-time case. Numer. Methods Partial Differ. Equ., 10, 129–149 (1994)
Huyakorn, P.S., Pinder, G.F.: Computational Methods in Surface Flow. Academic Press, New York (1983)
Murray, J.: Mathematical Biology, 2nd edn. Springer, New York (1993)
Squeff, M.C.J.: Superconvergence of mixed finite elements for parabolic equations. Math. Model. Numer. Anal. 21, 327–352 (1987)
Raviart, P.A., Thomas, J.M.: A mixed finite element method for 2nd order elliptic problems. In: Math. Aspects of the Finite Element Method. Lecture Notes in Math., vol. 606, pp. 292–315. Springer, Berlin (1977)
Thomée, V.: Galerkin Finite Element Methods for Parabolic Problems. Springer, Berlin (1997)
Wu, L., Allen, M.B.: A two-grid method for mixed finite element solution of reaction-diffusion equations. Numer. Methods Partial Differ. Equ. 15, 317–332 (1999)
Xu, J.: A novel two-grid method for semilinear equations. SIAM J. Sci. Comput. 15, 231–237 (1994)
Xu, J.: Two-grid discretization techniques for linear and non-linear PDEs. SIAM J. Numer. Anal. 33, 1759–1777 (1996)
Zhu, J., Zhang, Y.T., Stuart, A.N., Mark, A.: Application of discontinuous Galerkin methods for reaction diffusion systems in developmental biology. J. Sci. Comput. 40, 391–418 (2009)
Author information
Authors and Affiliations
Corresponding author
Rights and permissions
About this article
Cite this article
Chen, L., Chen, Y. Two-Grid Method for Nonlinear Reaction-Diffusion Equations by Mixed Finite Element Methods. J Sci Comput 49, 383–401 (2011). https://doi.org/10.1007/s10915-011-9469-3
Received:
Accepted:
Published:
Issue Date:
DOI: https://doi.org/10.1007/s10915-011-9469-3